Compression Ratio
An engine's compression ratio is a key factor in how the engine will
perform. Generally, engines with high compression ratio (10:1 and higher) have
less resistance to detonation and require high octane gas. Engines with
high compression ratios are generally not used in forced induction since
the margin for error is greatly reduced. Compression ratio is a fiercely
debated subject. This article is not about what compression ratio is "the
best"...it is about how to calculate it and how to achieve a desired
compression ratio.
On the surface, calculating your compression ratio seems like a very simple
task. You can find it using this formula:
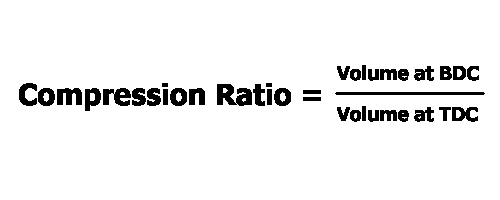
Unfortunately, it's just not that simple. As an example, let's look at two
Honda D series engines: the 88-91 D16A6 and the 86-87 D16A1. Both engines have a 75mm
bore and a 90mm stroke. They have approximately the same compression ratios
(9.1:1 for the D16A6 and 9.3:1 for the D16A1). You would think that inside,
they must be very similar and that maybe the D16A1's piston has a little more
dome to it? The combustion chambers and pistons in these engines are actually
substantially different.
D16A6 (88-91 CRX Si), 9.1:1 compression
D16A1 (86-87 Integra), 9.3:1 compression
As you can see, the Integra's combustion chamber is way bigger than
the CRX's combustion chamber. It's has over 15% more volume. However,
the Integra piston has a dome and the CRX piston has a dish. Simply put, the center of the Integra piston protrudes upward into the combustion
chamber. The center of the CRX piston does the opposite.
This is a graphical illustration of why it is a very bad idea to just call
a piston manufacturer up and say "I need 9:1 compression pistons for xyz engine.". Chances are, they have made certain assumptions that may or may not be true. For example, did they use a stock headgasket or a thicker one? What if I am not using the stock head?
To accurately calculate compression ratio, you must know several things:
1) The bore of the cylinder
2) The stroke of the cylinder
3) The volume of the combustion chamber
4) The compression height of the piston (Ask for this from the piston manufacturer)
5) The dome/dish volume of the piston (Ask for this from the piston manufacturer)
6) The piston-to-deck clearance (See this article on piston-to-deck clearance.)
7) The thickness of the headgasket
8) The bore of the headgasket
It sounds like a lot, but if you're going to do this right, do not skip out
on the details!! Know everything about the parts going into your engine. The actual formula for calcuting compression ratio based on the above variables is extremely long, complex, and leaves a lot of room for errors. Instead of trying to calculate by hand, I prefer to use C-SPEED Racing's excellent compression calculator.
Filling in the above, we have the following numbers for a stock 86-87 Integra.
1) Bore = 75mm
2) Stroke = 90mm
3) Combustion Chamber volume = 43.8cc
4) Compression Height = 1.181"
5) Dome = +1.5cc
6) Piston-to-Deck clearance = 0.000"
7) Headgasket thickness = 0.048"
8) Headgasket bore = 76mm
Plugging in the above numbers yields: 9.3:1 (as expected)
Now, let's take a look at my engine with Endyn's Rollerwave pistons. We have the following measurements:
1) Bore = 75.5mm (0.5mm oversized pistons)
2) Stroke = 90mm
3) Combustion Chamber volume = 43.8cc
4) Compression Height = 1.174" (stamped on the side of the box)
5) Dish = -6.25cc (given to me by Larry)
6) Piston-to-deck clearance = 0.006" (See article mentioned above)
7) Headgasket thickness = 0.040" (Cometic tri-layer steel gasket)
8) Headgasket bore = 76mm (per Cometic)
Plugging in the above numbers yields: 8.3:1
That compression ratio is a little too low for what I want to do. I'm aiming
for 8.8-9.0:1. This will spool the turbocharger faster and make off-boost driving more manageable. So what happens if I mill the head .030"? The easiest way to simulate this using the C-SPEED calculator is to decrease the thickness of the headgasket. In my engine, the effect of milling the head by .030" can be simulated by reducing the headgasket thickness from .040" to .010".
When I do this, I get that the compression ratio will be: 8.8:1
There are limits to how much you can mill your head, deck your block, or thin
out your headgasket. Generally, any combination of the 3 that results in a
loss of .040" or less is safe. However, to be sure, you must measure piston-to-valve clearance using clay (more on that later). Additionally, any combination of the 3 alters the camshaft timing in relation to the crankshaft. Adjustable camshaft gears (a common item on a performanc engine) can correct this.
Back to main







